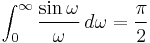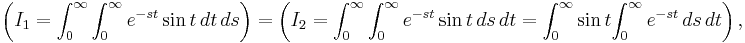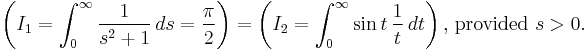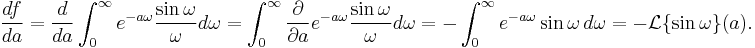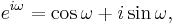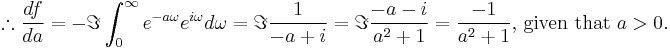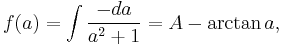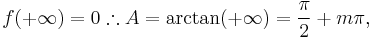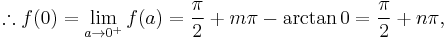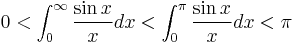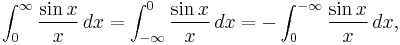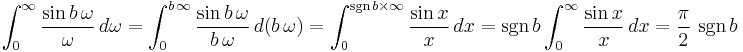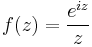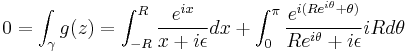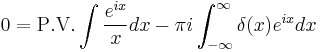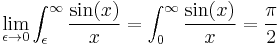Dirichlet integral
In mathematics, there are several integrals known as the Dirichlet integral, after the German mathematician Peter Gustav Lejeune Dirichlet.
One of those is
This can be derived from attempts to evaluate a double improper integral two different ways. It can also be derived using differentiation under the integral sign.
Contents |
Evaluation
Double Improper Integral Method
Pre-knowledge of properties of Laplace transforms allows us to evaluate this Dirichlet integral succinctly in the following manner:
This is equivalent to attempting to evaluate the same double definite integral in two different ways, by reversal of the order of integration, viz.,
Differentiation under the integral sign
First rewrite the integral as a function of variable  . Let
. Let
then we need to find 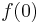 .
.
Differentiate with respect to  and apply the Leibniz Integral Rule to obtain:
and apply the Leibniz Integral Rule to obtain:
This integral was evaluated without proof, above, based on Laplace trasform tables; we derive it this time. It is made much simpler by recalling Euler's formula,
then,
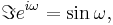 where
where 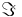 represents the imaginary part.
represents the imaginary part.
Integrating with respect to  :
:
where 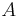 is a constant to be determined. As,
is a constant to be determined. As,
for some integers m & n. It is easy to show that 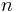 has to be zero, by analyzing easily observed bounds for this integral:
has to be zero, by analyzing easily observed bounds for this integral:
End of proof.
Extending this result further, with the introduction of another variable, first noting that 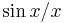 is an even function and therefore
is an even function and therefore
then:
Complex integration
The same result can be obtained via complex integration. Let's consider
As a function of the complex variable z, it has a simple pole at the origin, which prevents the application of Jordan's lemma, whose other hypotheses are satisfied. We shall then define a new function[1] g(z) as follows
The pole has been moved away from the real axis, so g(z) can be integrated along the semicircle of radius R centered at z=0 and closed on the real axis, then the limit 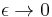 should be taken.
should be taken.
The complex integral is zero by the residue theorem, as there are no poles inside the integration path
The second term vanishes as R goes to infinity; for arbitrarily small 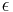 , the Sokhatsky–Weierstrass theorem applied to the first one yields
, the Sokhatsky–Weierstrass theorem applied to the first one yields
Where P.V. indicates Cauchy Principal Value. By taking the imaginary part on both sides and noting that 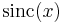 is even and by definition
is even and by definition  , we get the desired result
, we get the desired result
Notes
- ^ Appel, Walter. Mathematics for Physics and Physicists. Princeton University Press, 2007, p. 226.